{ Practical astronomy | Astronomy | The Moon | The lunar motion }
The lunar motion

The Moon shows different phases at different points of its orbit around the Earth, because only half of the Moon (and the Earth) are sunlit.
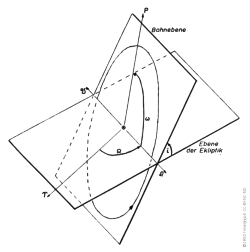
Like the Earth orbit around the Sun, the Moon's orbit around the Earth is approximately an ellipse, or a Kepler orbit. This orbit is five times more eccentric, but will still look very much circular. The first thing one would notice is that the Earth is not at the centre of the orbit, but set off to one side by 6% of the "radius". The points of minimum and maximum distance between the two bodies are galled the perigee and apogee, commonly as the apsides.
A more obvious measure of the position of the Moon in its orbit is the phase of the Moon: At New Moon the Moon is between Earth and Sun and none of the visible side is illuminated. At Full Moon the Earth is between Moon and Sun and all of the visible side is illuminated. In between are the First Quarter and Last Quarter, when the phase is a half Moon. In these constellations, the Earth-Moon line is sideways from the Sun-Earth line.
Regarding the Kepler orbit, on one hand we can try to approximate it by a circle, which is just the ellipse with eccentricity zero. On the other hand we can improve on the Kepler orbit by considering perturbative effects of the Sun and perhaps planets other than the Earth.
A circular orbit
Although a circular orbit is quite simple, we have to include the inclination i between the orbit of the Moon with respect to the ecliptic. With that goes the position of the intersection of the orbit and the ecliptic, the ascending node Ω:
i ≈ 5.15°
Ω(J2000) = 125.043355°
However, this is in practice not good enough. The first pertubative effect of the Sun we have to consider is nodal precession. Imagine the Earth-Moon system as a spinning bicycle wheel with the Earth on the axis and the Moon on the rim of the wheel. The Sun tugs at this object from an angle 5° outside the plane of the wheel. The typical spinning-top response is for the wheel to precess. Returning to the actual orbit of the Moon, this means that its nodes slowly move around the ecliptic. We then actually have
Ω(t) = 125.043355° − 0.05295392°/d (t − T0)
The rate of movement corresponds to a period of 18.61 yr.
In the presence of such rapid movement of the nodes, we parameterise time by the somewhat awkward mean longitude L. It is awkward, because it is the sum of two angles, first from the equinox along the ecliptic to the node, then from the node along the orbit to the Moon. The circular orbit approximating the Meeus orbit (see below) where the Moon has correct longitude at J2000 has
L(t) = 218.316133° + 13.17639653°/d (t − T0)
The daily motion corresponds to a period of 27.32 d, which is called the sidereal month.
Lunar standstill

Montage of how the Full Moon might have appeared over the recumbent of the Loanhead stone circle in Aberdeenshire on 2006-06-12 during major standstill. (Graphic from Cartes du Ciel, Chevalley 2019a).
I find the term standstill totally misleading. What is happening?
The ecliptic is inclined to the equator by about 23.5°. Hence the Sun, over the year, covers a declination range of ±23.5°. We expect something similar from the Moon, on a monthly basis, because its orbital plane is close to the ecliptic. However, there is about 5.2° inclination between the lunar orbit and the ecliptic.
At one extreme, if the lunar ascending node lines up with the vernal equinox, the Moon can cover a declination range of about ±29°. Due to the pertubative effect of the Sun, the nodes do slowly move along the ecliptic, meaning that such a lineup of nodes and equinoxes will actually happen, but the lineup is limited to a particular time.
That is what is called the major standstill. In the month when this happens, the Moon will exhibit the largest monthly declination change possible.
When the descending node aligns with the vernal equinox, the Moon stays closer to the equator than the ecliptic does. So the monthly declination range is only about ±18°. This is called the minor standstill.
The recumbent stone circles of northeast Scotland appear to be constructed with the major standstill in mind. The SSW part of the stone circle is realised with a "recumbent", a stone that lies on its side when all the others are standing. At major standstill the summer Full Moon stays very close to the horizon and can be seen to travel from culmination towards moon set just above the upper edge of the recumbent.
A Kepler orbit
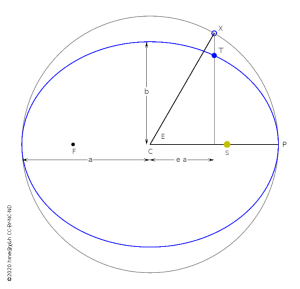
The elliptical Kepler orbit. For demonstration purposes, the eccentricity here is rather extreme at e = 0.6.
In the switch from a circular to an elliptical orbit we have non-zero eccentricity e and the extra parameter ω that describes where in the orbit the perigee is.
For the circular orbit we were not able to keep the ascending node Ω still, because perturbation from the Sun is just too strong. The same is the case for ω. The first thing that goes wrong with a Kepler ellipse is usually that it turns from a closed ellipse into a rosette-shaped, open path. We capture this issue by giving the perigee a slow movement. This is called apsidal precession.
Having the parameters Ω and ω we switch from mean longitude L to mean anomaly M to describe where in the orbit the Moon is. Both grow linearly with time by a rate n, which is the mean daily motion. The rate is different for M and L, because of the change in Ω and ω. Anyway, M is not an actual angle in space; it is just a measure of time. Instead of days it uses degrees, and its zero point is at perigee. By spying on the Meeus algorithm below, we get:
M(t) = 134.962914° + 13.06499245°/d (t − T0)
This daily motion corresponds to a period of 27.55 d, which is the average time from one perigee to the next and called the anomalistic month.
Spying again on Meeus algorithm, we find ω as
ω(t) = 318.309864° + 0.16435800°/d (t − T0)
The daily motion of ϖ=ω+Ω is 0.11140408°/d, corresponding to a period of 8.847 yr, the period of apsidal precession.
We can now write down the traditional Kepler elements, but including a gradual change of node Ω and apsides ω:
- a
- Semi-Major axis (in Gm). 0.3844 Gm.
- e
- Numeric eccentricity (dimensionless). 0.055.
- n
- Mean daily motion (in °/d). 13.06499245°/d, corresponding to an anomalistic month of 27.55455 d.
- ω
- Argument of perihelion (in °). 318.309864° + 0.16435800°/d (t−J2000). If we consider the rate for ϖ=ω+Ω, that is 0.11140408°/d and corresponds to a period of apsidal precession of 8.847 years.
- i
- Inclination (in °). 5.15°.
- Ω
- Longitude of ascending node (in °). 125.043355° − 0.05295392°/d (t−J2000). The rate corresponds to a period of nodal precession of 18.61 years.
The orbit integration is identical to that written down for the Kepler orbit of the Sun. We replace the Kepler elements as stated here and solve the Kepler equation, then shift and rotate the Cartesian coordinates according to a, e, ω, i and Ω.
Perturbation
The Sun has has a huge perturbing influence on the lunar motion. We have already accounted above for two effects:
- Since the Sun is 5° outside the orbital plane it tugs at the spinning top that is the Earth-Moon system to try and put the orbital axis upright. But the response is nodal precession, i.e. a change of the orientation of the orbit so that the nodes move along the ecliptic.
- Perturbations in general will cause the ellipse to be slightly inaccurate. The most obvious change possible is that the perigee moves slightly, changing the ellipse into a rosette. This is called apsidal precession.
These two effects have created an extra two definitions of the month, making it four in total:
- sidereal month:
- This is the orbital period, as one might observe against the backdrop of the stars. This is about 27.3 d.
- synodic month:
- This is similar to the sidereal month, but reference is made to the Sun instead of the stars. This is the most common definition of the month, the period from one New Moon to the next. This is about 29.5 d, considerably longer than the sidereal month.
- anomalistic month:
- Here the reference is not the New Moon, but the perigee. The time from one perigee to the next is about 27.6 d, only slightly longer than the sidereal month.
- draconic month:
- Here the reference is not the perigee, but the ascending node. The time from one passage through the ascending node to the next is about 27.2 d, slightly shorter than the sidereal month.
The three main perturbation effects of the Sun on the lunar motion are (Duffett-Smith 1981a, p.136ff; Wikipedia 2021b):
- Evection is an apparent change in eccentricity of the orbit. What the Sun does to the lunar orbit is vaguely similar to the tides that the Moon causes on Earth. For the Earth's sea surface imagine the lunar orbit, and for the Moon causing the tides imagine the Sun tugging more than average at New Moon and less than average at Full Moon. The orbit is stretched a little along the line from New to Full Moon (radial to the Sun) squashed a little along the line from First to Last Quarter (tangential to the Sun). Combine this with the ellipse having its long axis perigee to apogee, and the eccentricity will be more extreme when perigee or apogee line up with New or Full Moon. When they line up with First or Last Quarter, the eccentricity of the orbit is reduced.
- Variation takes account of the motion of the Moon in the solar gravitational field. At New Moon solar gravity is stronger than at Full Moon. The Moon speeds up toward Full and New Moon and slows down toward the Quarters.
- Annual equation is due to the variation in distance to the Sun. At perihelion the stronger solar gravity expands the lunar orbit. The lunar orbit grows in semi-major axis and in period, at aphelion it shrinks.
Perturbation after Meeus
The perturbation of the lunar motion, in particular by the Sun, is quite significant, and the algorithm to deal with this strays quite far from the idea of a near-eternal ellipse and its Kepler elements. The algorithm we use is taken from Meeus (1982a), but is goes back to lunar theory of the 19th or early 20th century; we will call it the Meeus algorithm here. Broadly, these are the steps:
- The algorithm starts by calculating six so-called elements as a
third-order polynomial in time, the standard epoch being
1900. These elements relate not only to the Moon, but also the
Sun and their relative positions. Some elements are familiar from
Kepler orbits. The six are:
- L': Lunar mean longitude. In our view, this is not so much a parameter of the orbit, but a parmeterisation of time. But in perturbation calculations it does take on more of an angle-on-the-sphere meaning.
- MSun: Solar mean anomaly. This could be calculated with our chosen algorithm for the movement of the Earth around the Sun, but this algorithm has its own determination of this parameter.
- M': Lunar mean anomaly. In a Kepler calculation this would be an alternative to L'; the difference being the argument of perihelion and longitude of ascending node. But here this takes on a slightly distinct meaning, vital for the perturbation calculations.
- D: Lunar elongation from the Sun.
- F: Lunar mean distance from the ascending node. In a Kepler calculation this would be an alternative to L' or M'.
- Ω: Longitude of the ascending node. This is one of the Kepler elements.
- a: Semi-Major axis. The present algorithm contains this implicitly in the calculation of the lunar parallax at time of observation. a is not a constant.
- e: Eccentricity. This is implicit in the application of perturbation terms to longitude, latitude and parallax. e is not a constant.
- n: Mean daily motion. This is part of the polynomial expression of M'. n is not a constant.
- ω: Argument of perihelion. In a Kepler orbit this would be L'−M'−Ω. In this algorithm it is not a constant.
- i: Inclination. This is implicit in the application of perturbation terms to latitude. i is not a constant.
- A few perturbation terms, expressed as periodic functions of time, are applied to the unperturbed elements. Another time parameter e (quadratic in time, not the eccentricity) is also calculated.
- The ecliptic longitude λ of the Moon is calculated by applying about fifty perturbation terms. These terms are periodic functions of combinations of MSun, M', D, and F.
- A similar calculation is made for the ecliptic latitude β, involving about 45 perturbation terms.
- A similar calculation is made for the parallax π (the apparent angular radius of the Earth as seen from the Moon). This is an inverse measure of distance. About thirty perturbation terms are involved.
- The distance is calculated as r = 6378.14 km / sin(π), using the equatorial radius of the Earth. (λ,β,r) are then converted into Cartesian coordinates (in the ecliptic of date, EOD).
The accuracy of the algorithm is about 10" in longitude and 20 km (0.006%) in distance.
Errors of the Kepler orbit
We can use the Meeus algorithm to "observe" the Moon and measure each month the distances at perigee and apogee (hence the eccentricity and semi-major axis) and the extreme latitude (i.e. the inclination).
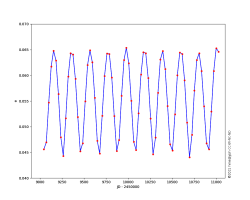
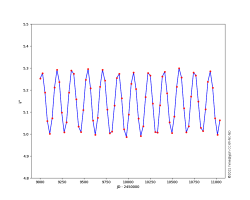
The first three plots show the orbit shape as measured from the "observation" of the Moon with the Meeus algorithm. For an interval of about 2000 d or 5.5 yr each month yields a red dot for each blue curve. The first plot shows the perigee distance q, the semi-major axis a, and the apogee distance Q. q shows the strongest variation and changes with a period of about 7.5 anomalistic months or 207 d. Q varies far less, with the same period and opposite phase, i.e. the apogee is most distant when the perigee is closest. The semi-major axis changes moderately, by perhaps 6000 km or 1.5% peak to peak around its mean value of 344000 km; the period is the same and the phase halfway between those of apogee and perigee.
The second plot shows the eccentricity for each anomalistic month. This changes considerably from about 0.045 to 0.065. The period is again 7.5 anomalistic months.
The third plot shows the inclination for each draconic month. It changes slightly by 0.3° peak to peak. The period is 6.33 draconic months or 172 d.
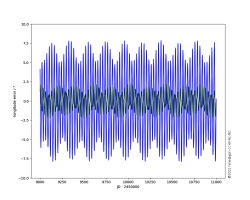
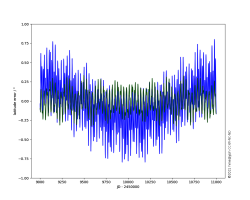
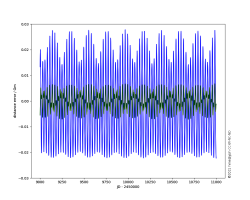
That experiment will not capture errors to do with the orientation of the nodes and apsides w.r.t. the ecliptic. These might show up in direct comparisons of longitude and latitude according to the circular or Kepler orbit versus the Meeus algorithm at various times. The remaining three plots show the errors of the circular (blue) and Kepler orbit (green) compared to the Meeus algorithm. The time interval is about the same as for the above experiment.
Ecliptic longitude λ is wrong on a monthly basis by up to ±10° for the circular orbit, and still ±2° for the Kepler orbit. Ecliptic latitude β is not quite as bad, with ±0.75° and ±0.25°.
Distance is wrong by ±25000 km for the circular orbit, a staggering ±6.5%. Again, the Kepler orbit does somewhat better at ±5000 km or ±1.3%.